Failure to understand simple mathematics although millions of dollars were spent on a tobacco and e-cigarette study
- Wednesday, 06 May 2015 23:02
By Dr Farsalinos
I am sure most of you are aware of the PATH study (Population Assessment of Tobacco and Health). This is a multimillion dollar survey (see slide 16, estimated at $117.4 million - big thanks to Brad Rodu for the information) of thousands of Americans, evaluating tobacco (and e-cigarette) use patterns. As everyone would expect, spending so much money (not even a dream for researchers in Europe) would mean that the study would be perfectly designed and verified repeatedly. However, I just realized that those who were assigned to prepare the survey questionnaire were unable to understand simple mathematics, simple even for a secondary school pupil.
To tell the story from the beginning, I was looking at an e-cigarette online survey, with one of the questions being the level of nicotine concentration liquids that participants use. The options had some peculiar concentrations, expressed in mg (instead of mg/mL) and % concentration. They were peculiar because the numbers did not match at all! I contacted the principle investigator of the survey and I was informed that the question was copied from the PATH study. I had a look at the questionnaire (page 91 of the pdf file) and here is the question asked:
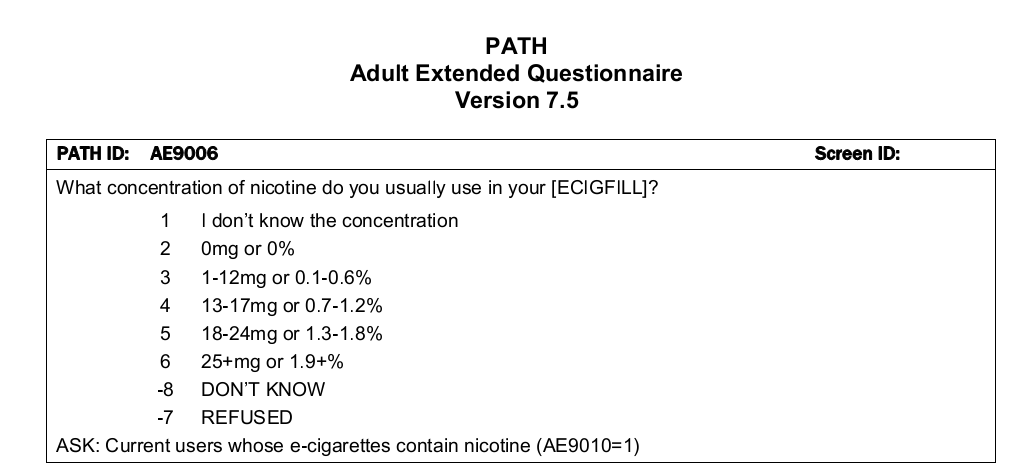
As everyone can realize, the concentrations in mg (mg/mL) and % simply do not match. To explain simple mathematics, mg/mL is in reality 0.00X g/ml, where X is the number of mg. Grams and mLs are of the same "magnitude", thus, 1 g/mL (1000 mg/mL) would be 100% concentration, while 1 mg/mL (0.001 g/mL) would be 0.1% (100/1000%) concentration. To convert mg/mL to % concentration, there is only one way to do it: convert mg to grams (= mg/1000) and then multiply by 100 (or divide mg/mL by 10)! It is that simple. Now, can anyone understand how is 1-12 mg (/mL) equal to 0.1-0.6% (which is basically 1-6 mg/mL – OK, 1 mg/mL matches with 0.1%)? How is 13-17 mg (/mL) equal to 0.7-1.2% (i.e. 7-12 mg/mL). The numbers do not match in any of the options provided (thankfully, 0 mg - /mL - is equal to 0%).
This is embarrassing for a study funded with millions. It is embarrassing for those who created the questionnaire and devised an unknown equation to match mg/mL with % concentration. If anyone understands how they made the conversion, please inform me (I would be very interested to determine how they did it). Another good lesson for all of us is to never trust anyone, even what we consider as the most credible source. In any case, I wonder how they will explain this mistake, and what will be the impact on the credibility of the study when such unexpected and embarrassing mistakes have been made.





